Canvas粒子系统:3D球体
发布于
shadow walker
前些日子在Codepen上看到了一个很惊艳的3D特效,一时惊叹,fork下来后,读了一下作者的源码,200多行,十分精简,但是内劲无穷。这里和大家分享一下作者的思路和一些基础的数学知识,
希望能给大家带来一点思考和启发,先来看一个效果:
动画生成过程
- 创建
canvas,设置canvas中心点,变量初始化:function setParameters - 生成指定数量的例子 同时完成例子的初始化(设置粒子
x,y,z以及增量vx,vy,vz):function createParticles - 初始化不同形状:
function setupFigure - 给
click(立即切换到下一图形) 和onmouseover(旋转), 绑定this因为addEventListener会把this绑定在DOM上:function reconstructMethod bindEvent给click(立即切换到下一图形) 和onmouseover(旋转) 绑定事件- 绘图:
function drawFigure
其中涉及坐标旋转的代码可以参照之前3D旋转球的博文 有详细说明 这里不做赘述:如何用Canvas做一个3D球
重点 (绘图: drawFigure)
球体制作在之前的博文里提到过,不做赘述.
对于环形制作,看看源码里的环形公式:
createTorus : function(){ var theta = Math.random() * Math.PI * 2, x = this.SCATTER_RADIUS + this.SCATTER_RADIUS / 6 * Math.cos(theta), y = this.SCATTER_RADIUS / 6 * Math.sin(theta), phi = Math.random() * Math.PI * 2;
return {
x : x * Math.cos(phi),
y : y,
z : x * Math.sin(phi),
hue : Math.round(phi / Math.PI * 30)
};
}
环形 x, y, z 推导 (x屏幕面的x方向, y屏幕面的y方向, z 垂直于屏幕面的方向)
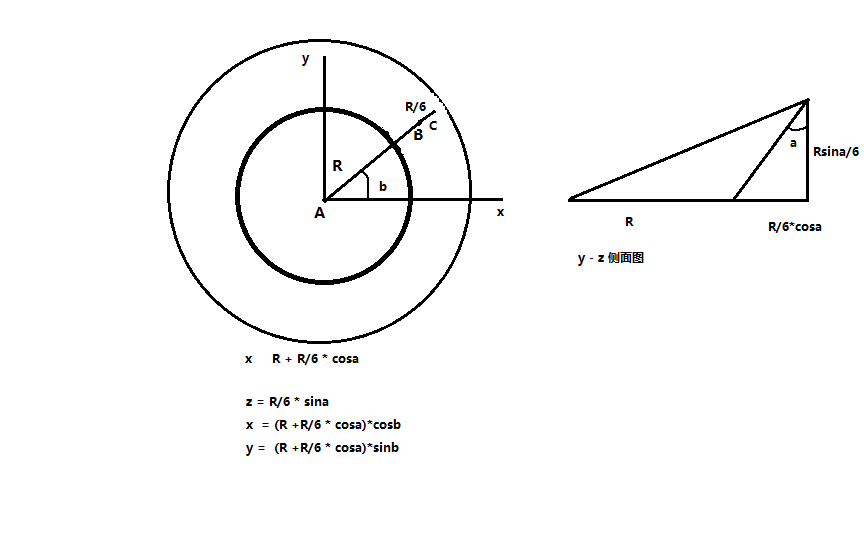
环形的z轴是相对屏幕对称的。
圆锥制作,看看源码里的公式
createCone : function(){
var status = Math.random() > 1 / 3,
x,
y,
phi = Math.random() * Math.PI * 2,
rate = Math.tan(30 / 180 * Math.PI) / this.CONE_ASPECT_RATIO;
if(status){
y = this.SCATTER_RADIUS * (1 - Math.random() * 2);
x = (this.SCATTER_RADIUS - y) * rate;
}else{
y = -this.SCATTER_RADIUS;
x = this.SCATTER_RADIUS * 2 * rate * Math.random();
}
return {
x : x * Math.cos(phi),
y : y,
z : x * Math.sin(phi),
hue : Math.round(phi / Math.PI * 30)
};
},
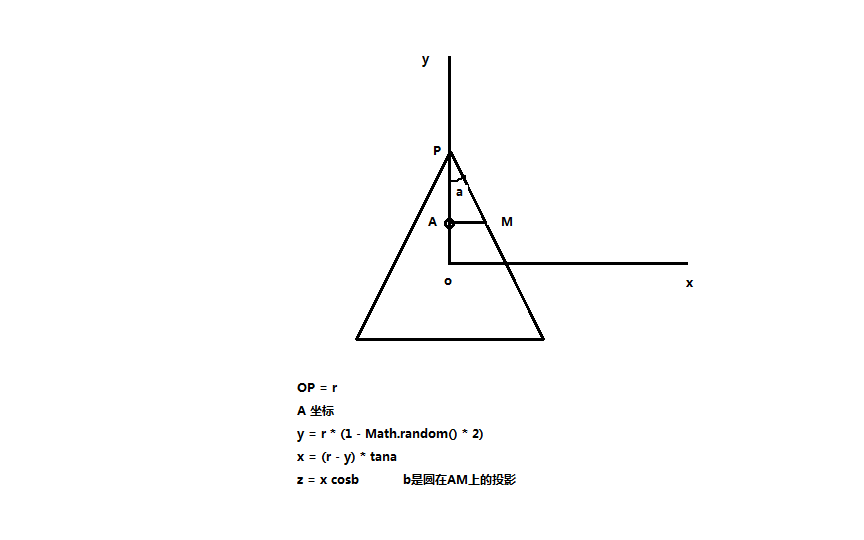
这里 tana 被作者替换成 1.5 tan30, 这里我们可以理解为a 为30,宽高比为1.5。
花瓶形(不知道数学里叫什么) 看看源码里的公式
createVase : function(){
var theta = Math.random() * Math.PI,
// x = Math.abs(this.SCATTER_RADIUS * Math.cos(theta) / 2) + this.SCATTER_RADIUS / 8,
x = Math.abs(this.SCATTER_RADIUS * Math.cos(theta) / 2) + this.SCATTER_RADIUS / 8,
y = this.SCATTER_RADIUS * Math.cos(theta) * 1.2,
phi = Math.random() * Math.PI * 2;
return {
x : x * Math.cos(phi),
y : y,
z : x * Math.sin(phi),
hue : Math.round(phi / Math.PI * 30)
};
}
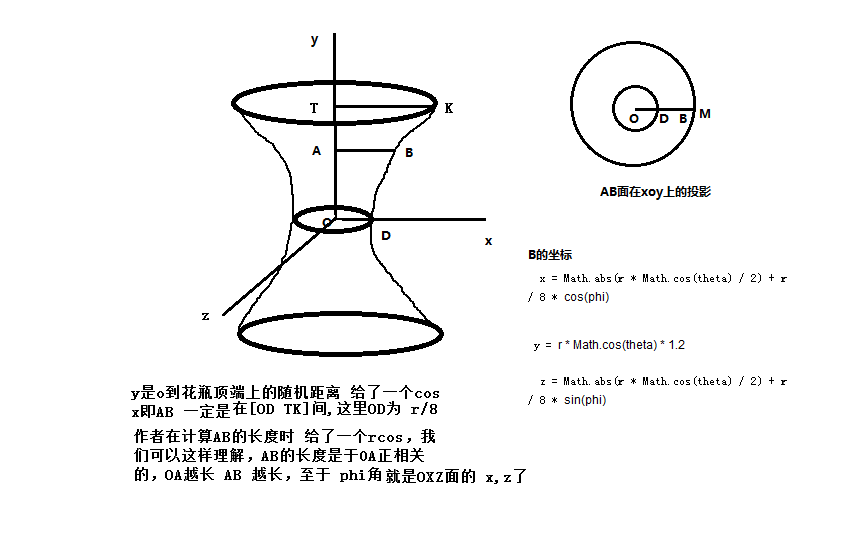
结语
Canvas 3D 坐标转换可谓基础,掌握好canvas坐标转换,配合图形的三维方程,可以让粒子做出美妙的运动,释放无穷尽的想象。
如需转载,烦请注明出处:https://www.fedev.cn/canvas/deformable-particles.htmlOff White X Max 90

